Order of operations (a mini-rant)

Order of operations is a major pet peeve of mine for two reasons:
(1) Some people love to use it in "gotcha" challenges to make other people feel bad about their math abilities. Here are some examples: facebook meme, a similar one, and this:

(2) For some students, it stands as a clear example of the idea that math is a set of arbitrary rules they have to accept and/or memorize (It isn't!)

I think there is a very different way of approaching this issue which is much more mathematically rich and fun.

1a. Multiple answers
The first and most obvious is to treat these memes as games and see how many answers you can justify by making the order of calculation explicit (use parentheses). Implicitly, this is the idea behind games like 24 or the traditional New Year's challenge (use the digits of the new year to make all values from 1 to 100).

2. Talk about history
Some launching questions: Where did the order of operations come from? Is it universally agreed?
This article from Tara Haelle does a good job of talking about this perspective and gives some further references.

3. Ask students for their own thinking
What do they think the order of operations should be? Why?
A tantalizing question: should there be an agreed (implicit) order of operations at all?

4. Talk about redundancy and error flagging (and error correcting) codes
Jordan Ellenberg's How Not to Be Wrong has a great discussion about redundancy in language and related code concepts. One key point is that we always face a trade-off between brevity and transmission errors. In other words, we can write short messages where every character carries critical, independent information, or we can use a system in which our messages are longer, but carry duplication and internal references that make our meaning more robustly clear. (compare the previous two sentences!)

Relying on a convention, like the order of operations, means that we can use fewer symbols to convey a mathematical expression. The great danger comes if the author and the reader don't share the same conventions! A less obvious danger is if a symbol gets garbled in the transmission, it may be hard to identify the error or even see that there was an error.

Some sort of number talks with J3

Based on conversations about the dots pictures from Math4Love:

Day 3
I notice

1. there's a number 3, but the number of dots isn't the same (it isn't 3)
2. five over here (pointing to dots) and zero on the down part (the bottom half of the 10 frame)
3. J0: I see some letters...
4. I even noticed that. I noticed there's this plus (points to dash - )
5. J0: I noticed this square
6. I noticed it was a line (bottom row of the 10 frame)
7. I noticed these triangles (the white space in 10 frame sections that have dots)
8. I noticed these are 5 and an extra one (on second page of day 3)
9. I noticed that there are four left (empty cells on second page)
10. J0: you saw 5+1, I see 2 + 4
11. Those two are together. The other ones are lonely.

I wonder

1. Why didn't they make 10 dots?
2. Why did they only cover the middle of the square (points to a dot in the upper left square of teh 10 frame)?
3. I wonder, how do numbers talk? (after I read the title of the slide to her)
4. I wonder, why do they only put 1 on the bottom row?
5. I wonder, can we arrange them so none are lonely

Day 4

I notice

1. Five on the top and five on the bottom
2. Ten
3. five and four, nine

Day 5

I notice

1. This doesn't have a box to go in (a 10 frame)
2. It has a dot in the middle
3. we can count them 2, 2, 2 (pairing them up)
4. if we take 2 away, there will be four
5. the sides are the same (it has a line of symmetry in the middle)
6. it looks like an animals footprint
7. the top four make a diamond
8. If we turn our body to the side, the top four make a rectangle
9. taking out the two in the middle, we have a square
10. it has 8 dots.
11. the number of dots doesn't match the day number

I wonder

1. is it a real footprint?
2. I wonder, if we take the bottom five, it would be 3?

Solving contest problems (challenge from Mike Lawler)

These are my notes working through problems posted by Mike Lawler on his blog. You'll have to go there to see the problem statements.

The intended value of this write-up is to show examples of the actual problem solving thought process someone has followed, not just a polished solution.

Problem 19
Since the circle has area 156 π the radius squared is 156, which is 4 * 3* 13. That seems like a strange number, I'm curious to see where it will make calculations come out nicely, later in the problem.

We're told OA has length 4 sqrt(3). Squaring that only gives 48, so A is well within the circle. That means triangle ABC has vertex pointing toward O along the perpendicular bisector of side BC.

This lets me set up a picture of a right triangle with legs length $x$ (half the side of the equilateral triangle), $x$ sqrt(3) + 4 sqrt(3) (the altitude of the equilateral triangle plus OA) and hypotenuse r (2 sqrt(3*13)).

Applying the pythagorean theorem and simplifying along the way:

$$x^2 + 3(x+4)^2 = 156$$
$$4x^2 + 24x + 48 - 156 = 0$$
$$x^2 + 6x - 27 = 0$$

Visually factoring gets me $x$ is either -9 or 3.  3 is much more reasonable for half the length of the side of a triangle, so my answer is 6.

Note: If this weren't a contest problem, I might try to think about the -9 root a little more carefully.

Equiangular hexagon
I had already solved this problem from a recent tweet of Mikes, so I can't fully recreate my thought process.  Here were some of the highlights:

• wonder why 70%
• draw a picture: fail to notice that triangle ACE is equilateral
• Split the hexagon into two trapezoids by line CF
• Calculate the area of those two trapezoids
• Calculate the length from C the intersection with AE and segment CF.
• Calculate the area of ACE based on the two subtriangles split by CF.
• Obtain the quadratic equation for r based on the formulae for the two areas and the given 70% parameter.

School Competition

Let's call Andrea's rank $m$ for median. Since she is the unique median, there must be $2m - 1$ total contestants. We also know:

• $m \leq 36$ because Andrea scored higher than Beth who was ranked 37th
• $64 \leq 2m -1 $ because Carla ranked 64th, so there were at least that many competitors
• $3 \mid (2m - 1)$ since every school sent three competitors

The first two inequalities tell us that $33 \leq m \leq 36$. Because $2m -1$ is a multiple of three, $m$ can't be a multiple of 3, so it has to be 34 or 35. Calculating mod 3, we can quickly check both and see that $m$ has to be 35.

That means the competition had 69 competitors from 23 schools.

Using multiple choice
After putting together these notes, I saw a commenter on Mike's blog use "solution by multiple choice." When I was doing timed tests/contests, I would make use of the options as part of my strategy. However, I don't do that now, since I'm much more interested in exploring the mathematics of the problems than getting the final answer.

Leftorvers with 100 game

In Grades 3 and 4, we played a nice game that (I think) we got from Marilyn Burns. Looking for a reference after the fact, I see it explained in her book Lessons for Extending Division.

Basic play

• Start with a target number (we used 100) and collection of available divisors (we used integers 1 to 20)
• Players take turns choosing a divisor from the remaining available options. They divide the current target by that divisor and keep the remainder as their score for the turn. They also subtract the remainder from the target to create a new target for the next player.
• Each divisor gets crossed out when it is used, so it can only be used once.
• The game ends when the target is reduced to 0 or when all available divisors are exhausted.
• We played as a two player game.

Here's an example of a game play:

Player 1 chooses 17. 100 = 17 * 5 + 15, so player 1 scores 15 points, the target is reduced to 85, and 17 is no longer available as a divisor.

Player 2 chooses 20. 85 = 20 * 4 + 5, so player 2 scores 5 points, the target is reduced to 80, and 20 is no longer available as a divisor.

Player 1 chooses 14. 80 = 14 * 5 + 10, so player 1 scores 10 points, the target is reduced to 70, and 14 is no longer available as a divisor.

Player 2 chooses 18. 70 = 18 * 3 + 16, so player 2 scores 16 points, the target is reduced to 54, and 18 is no longer available as a divisor.

Player 1 chooses 19. 54 = 19 * 2 + 16, so player 1 scores 16 points, the target is reduced to 38, and 19 is no longer available as a divisor.

Player 2 chooses 13. 28 = 13 * 2 + 12, so player 2 scores 12 points, the target is reduced to 26, and 13 is no longer available as a divisor.

Player 1 chooses 15. 26 = 15 * 1 + 11, so player 1 scores 11 points, the target is reduced to 15, and 15 is no longer available as a divisor.

Player 2 chooses 16. 15 = 16 * 0 + 15, so player 2 scores 15 points, the target is reduced to 0 and the game ends.

Player one wins 52 to 48.

Our experience

We found this to be a fun, interesting, and engaging game. The practice with dividing and remainders was pretty obvious. In addition, it opened up some opportunities for strategic thinking, particularly at the end-stage of the game. I think there are also several good extension explorations.

Extensions

First, I created a simple pencilcode program for two players to play this game against each other. Here's a playable version (and here's the code).

Second, you'll notice that the first player in our sample game followed a "greedy strategy."  At each stage, that player chose the divisor that would give the most points on that turn. If you look closely, that isn't the best strategy at the end of the game.

So, a natural exploration is to find the best strategy for different starting targets. One specific point of about which we're curious: is it ever desirable to skip your turn (choosing 1 as the divisor is effectively a turn skip)? 

Some other areas for investigation:

• must the game always end on 0 or can we run out of divisors?
• given a target and collection of starting divisors, what is the shortest (number of turns) game possible? What is the longest game (number of turns) that does end at 0?

Equilateral Triangles Puzzle (from Twitter)

Mike Lawler issued a call to respond to a nice challenge Matt Enlow posted on twitter:

Equilateral ΔABC inscribed in a circle. 2nd equilateral ΔPQR such that P is on segment AB and Q & R are on arc AB. Locus of center of ΔPQR?

— Matt Enlow (@CmonMattTHINK) November 17, 2016

My scribbles

I will admit that, initially, I had no good ideas about how to approach this puzzle. Intuitively, I was drawn to the idea of specifying the point P on the chord AB, building the equilateral triangle PQR, and then finding the center of that triangle. In the spirit of doing something, I decided to set up a coordinate system and think about the resulting equations:

Now, these are a mess and didn't really go anywhere, but, I had two thoughts as I was writing these out:

1. Since we are just intersecting lines and circles, we are probably looking for a conic section
2. The fact that triangle ABC is equilateral gives us a relationship between the side length and the radius of the largest circle, but I doubt whether it is critical for this problem. My suspicion is that we will get something similar for any chord.

Now, I'd had the suspicion that the coordinates for the center of an equilateral triangle was the average of the coordinates of the vertices, so I did a little work to confirm that:

At this point, I felt that I wasn't really making progress. In particular, I felt that I didn't really have the right ideas about how to find Q and R, given P.

I turned to geogebra to build the picture. Given Matt's request not to send screenshots, I felt that this was a bit of a cheat, but anyway...

I still didn't have a good idea about how to find Q and R, so I first built a worksheet where Q was a free point, from which R would be constructed so that the triangle PQR is equilateral. Then, I played around with Q until it and R sat on the original circle.

After doing this, I had two observations/conjectures. Taking C as the center of the original circle:

1. we know the angle CPQ is 150 degrees
2. we know that the line CP is the perpendicular bisector of segment QR

Neither is really tricky, so I was a bit chagrined to have missed them earlier, particularly the second point.

With that I now had a method to (a) draw more realistic diagrams and (b) reduce my Geogebra sheet so that only P's location on AB was an open degree of freedom. Here's a link to that Geogebra work.

Where I am now

Playing around with the Geogebra sheet, I made and tested conjectures about what conic shape could describe the locus. For simplicity, I hoped it was a circle, but testing three points shows that doesn't look right. Instead, it looks like a hyperbola. With that, my plan is to return to the cartesian plane, define a friendly reference variable, and check that I get the right relationship for the coordinates of our center points.

Geogebra FTW

Putting aside the fun of working on a nice problem, this served me as a great reminder about Geogebra. As a result, we'll soon be playing Euclid the Game at home with the kids.

Politics, math, and dog-whistles

In Frank Herbert's Dune, there is a cool idea of a military language with a flexible structure so that any pair of people can speak to each other in a way that they will understand, but which no listener will understand.

Modern politics and social media are moving closer to realizing this idea, through dog-whistles.

For example, I was really struck by this ad for a math curriculum package (see the second paragraph):

For the author and their intended audience, Common Core means something very particular and particularly bad.

Personally, I find the fragmentation of language very troublesome. Among other things, it contributes to a certain type of magical/fallacious thinking, nicely exemplified by the popularity of ObamaCare (according to some survey results):

1. The Affordable Care Act gets broader support than ObamaCare (they are two names for the same thing)
2. Individual provisions get significant majority (more than 50% of respondents) support, while ObamaCare does not only earns minority (less than 50%) support.

This first point is silly, and I don't see any logical way to redeem that combination of beliefs. For the second point, while it is possible to logically reconcile the two observations, the most likely explanation is that people surveyed were mis- or under-informed.

Puzzling puzzlers

Preparing for classes today, we went through our lists and links to gather a list of puzzles for the kids. Hopefully, this will help short circuit the work we have to do next time.

• Eric Friedman Puzzle Collection (highly recommended by Mathpickle)
• Cryptarithm: shape substitution version, basic ones, 
• Funny dice 
• SolveMe Mobiles
• Make your own coin system
• Multiplication: Miller's Puzzle from Dudeney
• Find the Factors
• Multiples of 7 and asymmetric information: What's my number from Tanya Khovanova
• Nonagrams and battleship puzzles
• Logic Grid puzzles: Two dimensional starters, Logic-Puzzles, 
• Distortions logic puzzle (nice twist on truth tellers or liars)
• Questions from an adult, general public, math contest: Math Mama Writes
• Math is Fun collection
• Of course, there are a lot of great puzzles on Mathpickle
• Sudoku
• Number arrangements: magic squares, "magic" triangles and stars
• Zukei geometry puzzles (find the "hidden" shape)
• Area maze, K-maze, B-cross, Shikaku, Dokoeq (all from Naoki Inaba)
• Verruca Values

Election analysis

This isn't exactly a kids-learning post, so apologies to anyone disappointed. This is my attempt to organize some thoughts and analysis around the results of the 2016 US election. The questions I'm posing are "Why did Trump win/Clinton lose the presidential election?" and "What are the lessons for 2018 and 2020?"

This analysis is not complete, so apologies to anyone who wants a nicely packaged story.

I welcome data, analysis, and different perspectives supported by evidence.

Range of theories

CNN starts us off with a nice collection in their article: 24 theories why Trump won.

Before I summarize their list, I'll note that I intentionally use the combination "Trump won/Clinton lost" rather than focus on a single side. What I'm thinking is that there are factors specific to both sides as well as relative factors at play. It isn't appropriate to look at one group in isolation. That idea helps me with a simple taxonomy of their theories:

Trump-focused theories

1. Media. There are several sub-theories: (a) social media and fake new echo chamber: Trump supporters were ill-informed because of systematic problems with modern media. Facebook is particularly cited as a key culprit. (b) celebrity plays stronger than substance, 
2. Trump appealed to white males, so they supported him. Subtheories are (a) xenophobia/racism/sexism, (b) backlash to political correctness.
3. Trump appealed to voters who have been struggling economically

Clinton-focused theories

1. Voter suppression directed toward minority voters who would typically support the Democratic candidate. 
2. Leaked information related to scandals. Some sub-theories: (a) Russia provided hacked information, (b) FBI.
3. Third party candidates drained support from Clinton.
4. Clinton was a weak candidate who did not appeal sufficiently to those who formerly voted for Obama (a) saddled with too much negative baggage (b) not properly tested or vetted through the primary process

Relative theories

1. anti-establishment fervor

The most popular story

My impression is that the most popular theory is Trump 3: Trump won the votes of those who are struggling economically. One strong example from before the election is the Guardian's My Journeys in Trumpland.

Now, empathy is good and I applaud people trying to understand each other. That said, is the economic plight of the non-elite white voter the reason for this election outcome? It is a popular story with a nice human/humanizing face, but doesn’t ring true to me. Here are the reasons I’m skeptical.
First, from my own experience, this group has been struggling since the 1980s (maybe earlier). The story is not new.

Second, it looks like Trump’s overall support was about what we should expect from a “generic republican,” maybe underperforming a little. I’m basing this on the comparison against the past two elections (see here for example).

Third, the evidence that Trump’s supporters (on average, whatever that means) are fairly well-off (see 538 primaries and the income table from CNN exit polls.)

Confusion about voter turn-out

I am currently confused about voter turn-out. These articles from CNN and 538 seem contradictory, but I haven't had a chance to work through their numbers and reconcile.

Anti-establishment

Perhaps one useful test here is the degree to which incumbents were re-elected compared with past elections. I haven't yet gathered the statistics for congressional elections, but my impression is that incumbents were overwhelmingly re-elected, basically in line with past experience, if not more. See how few districts and states are cross-hatched in these maps, (marking that shows a flip in the party controlling that seat):

2016 House of Representatives results from NY Times as of 14 Nov 2016

2016 Senate results from NY Times as of 14 Nov 2016

Multiplication & Fractions Math Games from Denise Gaskins (a review)

I really like Denise Gaskins's new book: Multiplication & Fractions Math Games (links to paperback edition and accompanying printable.) How much do I like it? Well, I had already written a lengthy review that, somehow, I managed to lose and am now back writing another one.

I'm going to forego my preferred Good (what I liked), Bad (arguable weaknesses) and Ugly (unforgivable sins) because I don't really have anything to say in those two negative categories. Instead, let me just talk about who would find the book useful and why.

Group 1: Parents who feel their own math skills are weak.

Maybe you never really understood what multiplication means or what fractions are? As long as you start with an open mind and are willing to engage playfully, the activities in the book can help you as you help your kids. It starts with models that are visual explanations of the concepts. Gaskins also breaks learning these concepts into comfortable steps that emphasize patterns and relationships, the real ideas that are behind properly understanding multiplication and fractions (indeed, math generally). The sequence of games in each section starts by building familiarity and then fluency (speed) to solidify all of that work.

Group 2: Parents who worry about their kids struggling with these concepts

Anecdotally, these two areas are the first major stumbling point for students in their math studies. As I noted above for parents themselves, the sequencing in the book will help kids develop a strong foundation, beginning by understanding what multiplication means (and what fractions are). Beyond that, playing the games will make these concepts familiar and, I believe, lead them to recognize examples around them in their daily lives.

Group 3: Families who like to play games

Kids (and parents!) find these games fun. I've been field testing math games for the last 18 months and keep seeing how engaged kids get when playing math games. I have played many, though not all, the games in Multiplication & Fractions and strongly believe the games in the book will be winners with most kids.
Now, let's face it, you might not be thrilled with every game. For example, I wasn't so excited by the idea of playing War variations. However, a lot of other games in the book that are strategically and mathematically rich. Also, truth be told, my kids and students have really enjoyed playing multiplication war!

So, there's really nothing weak in this book?

There is only one worry I have about making a blanket recommendation: parents who start with a completely wrong mindset. If you believe in speed over understanding or mathematical gifts instead of effort, then this book is the wrong place to start. Instead, read Dweck's Mindsets and spend time with Jo Boaler's website. Maybe also re-watch Karate Kid (no joke, this is what I'm currently playing.)

A disclaimer, sort of

I'm friends with Denise Gaskins and got a review copy of this book. However, you should understand that we're friends because I'm a fan of her math teaching work and not the other way around. We've never even met in real life and, in fact, live in different continents. I know of her because advocacy of play-based math learning. I admire her because she is one of the best at creating resources that bring this material into the reach of the typical parent.

Vacation Plan: Emotional Skills

This month is a school vacation period for the three Js. One area of focus this month will be on emotional intelligence skills.

Component Skills

We found a nice overview on Psych Central. We talked through the first four with J1 and J2 to start the month:

1. Self-awareness: (a) recognize your own emotions and their effects, (b) sureness about your self-worth and capabilities
2. Self-regulation: using a number of techniques to alleviate negative emotions
3. Motivation: tools to manage motivation to achieve goals.
4. Empathy: discerning the feelings behind others’ signals

The article also includes Social Skills as a category, but these seem separate to us.

Around the discussion of empathy, J1 asked how it differs from sympathy. We think that a difference is understanding how other people feel and their perspective (empathy) vs sharing their feeling (sympathy). I admitted that I do not have much sympathy.

A vocabulary list

Underlying many of these skills is a vocabulary of emotions. We found two nice resources for this:

• Emotions color wheel: this is a great visual for the kids. 
• Vocabulary list for greater shading: the idea is to move beyond the standards, happy, sad, angry, to get more shading and nuance. Another hope is that, in the moment of analyzing the emotion and comparing with the vocabulary, it will help their self-awareness and provide a point of detachment from the emotion.

Our Focus

J1 chose to focus on skills relating to empathy and sympathy.

J2 chose to focus on skills related to self-regulation.

Daily Schedule

Supporting this skill development and general household organization, we are posted this schedule for the month. You can probably tell that the boys helped write the schedule:

Things to do everyday:

1. 3 pages of Beast Academy and discussion with J0
2. 10 minutes of spelling with P
3. Vocabulary: writing a sentence and 5x words (5 words/ day for J1, 3 words/day for J2) 

07:00 Wake up
          urinate
          put dirty nightclothes in basket
          shower
          get dressed

08:00 prepare breakfast
          eat breakfast
          brush teeth
          poop

12:00 help with lunch
          eat lunch
          clear table from lunch

17:00 help with dinner
          eat dinner
18:30 clear table from dinner
          practice music
          put dirty clothes in basket
          urinate
          bath
          brush teeth

20:00 get in bed
          listen to story
20:30 lights out

Block blobs redux

Last December, we played Block Blobs (notes here). This week, we are trying a slightly modified version for two digit multiplication.

The Game

Materials

• 4d6. Two dice are re-labelled 0, 1, 1, 2, 2, 3 (see notes below)
• Graph paper (we are using paper that is roughly 20 cm x 28 cm, lines about 0.5 cm apart)
• colored pencils

Taking a turn

Roll all four dice. Form two 2-digit numbers using the standard dice as ones digits. Then, use your color to outline and shade a rectangle in the grid so that:

1. the side lengths are the 2-digit numbers you formed with the dice
2. At least one unit of the rectangle's border is on the border of your block blob
3. Note: the first player on their first turn must have a corner of their rectangle on the vertex at the center of the grid. The second player has a free play on their first turn.
4. Write down the area of your rectangle

Ending the game

The game ends when one player can't place a rectangle of the required dimensions legally.

When that happens, add up the area of your block blob. Higher value wins.

Notes

"Counting" sides
The side lengths of the rectangles are long enough that counting on the graph paper will be irritating. Instead of counting directly, they can measure the side lengths. For our graph paper, the link between the measurement and the count is nice, since the paper is very nearly 5mm ruled, so they just double the measure. I think this is a really nice measurement and doubling practice, too.

Dice labels

Other labels could be used on the special dice. We chose this arrangement because of the size of the graph paper. Rectangles with sides longer than 40 often won't fit and we think we will even need some single digit rectangles to allow a fun game length. An alternative we are considering is 0, 0, 1, 1, 2, 2.

As an alternative to labeling the dice with new numbers, you could label them with colored dots and give out a mapping table. For example:

blue corresponds to 3
red corresponds to 2
green corresponds to 2
yellow corresponds to 1
black corresponds to 1
white corresponds to 0

This would keep the tens digit dice distinct from the ones digit dice and allow rapid modification if you want to change the allowed tens digits (just tell everyone a new mapping). Alternatively, you could create a mapping using "raw" dice and even allow more strategic flexibility from the players. I have a feeling that this would be confusing to most kids, though.

Reinforcing the distributive law

To facilitate calculating the area and reinforce the distributive law, you might have the students split their rectangles into two (or four or more) pieces and calculate the partial products. You can further decide whether to ask them to split the sides in particular ways or encourage them to find the easiest way to split to help them calculate.

Our math curriculum

Sasha Fradkin (who writes one of our favorite blogs) asked a question about the curriculum we use. My reply was getting long, so I decided to make it into a separate post.

Do we use an existing curriculum or are we making our own?

We are doing a mix. My wife prefers to have a linear curriculum as a guide and fall-back, in case there wasn't time to plan anything more customized. She currently uses:

• RightStart/Abacus Math: ok, but not exceptional curriculum, highlight is the extensive use of physical manipulatives.  
• Beast Academy workbooks: wrote more extensively about this in a review before. I think these create good jumping-off points for really fun conversations. 
• DreamBox: for consolidation of standard skills, our enthusiasm for this is waning, rather than waxing right now (noted in same review as BA). 

We also use the CCSS math standards as a reference. I periodically check against the standards to see whether we are missing anything. If so, I will go to the Georgia Standards of Excellence, read through their activities for the related unit, and pick a couple that seem fun.

If I were forced to use only a single source, GSE would be my recommendation.

About Georgia Standards of Excellence
As far as K-5 math, this is a really awesome resource with a ton of great activities. For some reason, we find the webpage organization a bit confusing, so here is our quick recipe.  

To get to the great activities, I click the expansion menu in the right-hand box for the grade level of interest, then look at the curriculum map for that grade. I find the topic of interest, then click the link for the unit that covers that topic. The unit doc includes a lot of teacher background, which I mostly skip and focus on the activity descriptions.

Games and explorations
My personal preference is much less structured. I really like games and explorations and spend a lot of time exploring math activities on the MTBoS. Most of what I do with the kids is inspired by something I saw while doing my own play.

That said, there are some sources that are so good, we are essentially going through all of their activities:

1. Mathpickle. Cannot say enough positive about this.
2. Peter Liljedah's numeracy activities. Now that I notice them, I bet his good problems, card tricks, and resources pages will all have gems as well. 
3. NRICH and Wild Maths.

Teaching goals for English Language Arts

These are our current objectives for English language study with our children. These are very high level goals, but we feel it is important to write these down so that they can guide our detailed choices. Ultimately, our hope is to guide the kids to be independent learners.

Strong Readers (in-bound communication)

• Able to use reading as a tool for further learning. This means they must:
• Enjoy reading
• Have a large vocabulary and tools to build their vocabulary
• Good comprehension and tools to analyze what they are reading
• Familiarity with sources of information
• Read a wide variety of material: topics, authors, styles, forms

Writing (out-bound communication)

• Able to communicate ideas clearly and effectively (writing and speaking)
• Comfortable with the mechanics of writing: vocabulary, spelling, grammar, punctuation, physical writing and typing
• Learn a writing process: research, brainstorming, forming ideas, organizing ideas, drafting, revising

Conduit for other content

We will make use of language activities that also teach them:

• History: having data of history to learn from the past, ideas of historiography and perspective
• Science: technical jargon, tools to understand and develop scientific frameworks
• Philosophy and comparative religion: what are the great questions, different perspectives, forming their own values and understanding those of other people
• Current events: understanding the current context of their lives

Develop skills that support other language learning

• Grammar frameworks
• Methods for learning vocabulary
• Motivation

A talk for parents about math at our school

Today, P led a session for the other parents at the school. We wanted to share the material and some links for those who weren't able to attend.

Agenda:

1. What does it mean to be good at math? What are we trying to achieve?
2. Key concepts we are using in teaching: Concrete-Pictorial-Abstract, Concept Progressions
3. Examples
4. What should parents do at home?

Good at Math

To start, P asked the parents, what does it mean to be good at math? Some of the answers:

• can add, subtract, multiply and divide
• calculate quickly
• able to estimate 

The range of opinions was good to see. Many ideas fell within the traditional answer: being good at math means being able to calculate precisely and quickly. We were especially pleased to hear skill at estimating as one of the ideas.

Our additions:

• Thinking logically. For example, if a certain thing is true, what else is true? 
• Looking for patterns and relationships; forming connections with other things they know.
• Asking questions about what they see, especially investigating structure
• Persevering

Admittedly, these are necessary for many other subjects. Math is a particularly good place to develop these skills because there is much greater objectivity and right/wrong are often clearly distinct. In this domain, the power of reasoning and independent thought is stronger than the power of authority.

Process of Learning Math

For this discussion, we focus on two key concepts in the way we teach and study math: (a) the Concrete-Pictorial-Abstract modes and (b) multiple models in progression and contrast.

C-P-A

"Concrete" means using physical objects. For example, a pile of 15 beads can be a concrete representation of the number 15. Taking 2 beads in the left hand and 3 in the right hand, then combining them is a concrete experience of adding 2 and 3.

In this mode, children are able to see, touch, move, examine, smell, and hear mathematics.  

"Pictorial" shifts to pictures on the page or board. For example, a picture of a room showing a vase with 2 flowers and another vase with 3 flowers can lead us to identify 5 flowers all together.

In this mode, children are able to see, construct (by drawing themselves), obliterate (by crossing out), and add color (by coloring, naturally) the mathematical objects.

"Abstract" is where we shift to symbols. For example, 2 + 3 = 5. This is a sequence of 5 symbols that don't offer any clues to their own meaning.  

In this mode, children are able to imagine and to move beyond physical constraints or necessities.

When new concepts are introduced, they generally go through each stage, starting with concrete, then pictorial, then abstract. This doesn't mean that abstract is superior, however. The ability to move back and forth, to give specific examples, to draw diagrams, to demonstrate a concrete model is also very important.

Multiple Models

Complementing the three modes, we also try to have multiple models, different ways of seeing, new concepts. There are two great resources that nicely illustrate this.  First, the models of multiplication posters from Natural Math:

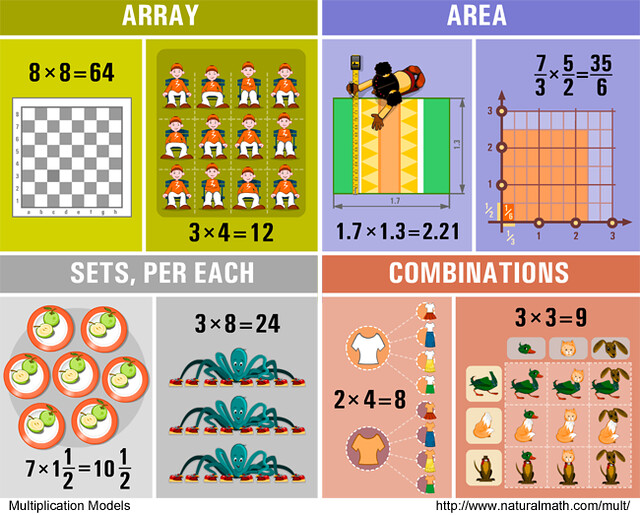4 of 12 models at Natural Maths

For the discussion, P gave examples of the equal groups model (sets per each), repeated addition, array, number line, and area.

I strongly encourage you to take a look at all 12 models in their poster, so here's the link again:
http://naturalmath.com/2013/09/12-models-of-multiplication/

The second resource is Graham Fletcher's series of progressions videos: Addition and Subtraction,
Multiplication, and Division.

For this talk, we presented abridged versions of the content in the multiplication and division videos. For your ease and viewing pleasure, here they are.

and the division video:



What to do at home
1) Ask questions
Parents can relax about being the source of knowledge. Don't worry about "teaching" or having the right answer. Instead, develop habits to cue thinking and their use of problem solving strategies:

• How do you know?
• What pictures could help us?
• What do you notice? What do you wonder? This works especially well if the parent serves as scribe writing down the kids' ideas.

"How do you know?" does three things. First, it is one way to escape from the child's questions "is this right?" Remember, the power of reasoning is stronger than the power of authority. We want to reinforce this by side-stepping calls to authority.

Second, it is a cue to get them thinking about their own thought process, which aids learning.

Third, this opens a potential discussion about different ways to attack the problem. Comparing and contrasting multiple strategies is a powerful tool for deeper learning.

"What pictures could help us?" is a cue to move between the Concrete-Pictorial-Abstract modes. If available, go to Concrete by asking about objects or physical models that are related.

"Notice & wonder" is a deep topic. One key idea is that, by serving as the scribe, we parents demonstrate that we care about the ideas that the kids have, we know they can contribute to solving the problem. This also gets us listening and understanding their perspective.

Notice & Wonder also involves skills that the kids will strengthen with practice, starting with superficial or (mathematically) irrelevant ideas and eventually moving on to thoughts about patterns and structure.

 For more about notice and wonder, please take a look at Annie Fetter's talk, 

2) Talking numbers to develop number sense
Two examples of number sense. Say we bought 8 bags of snacks and each bag cost 17 baht:

• I know that the total cost is not 1000 baht based on understanding order of magnitude.
• I know that the total cost is not 137 baht based on the pattern that all multiples of 8 are even

Like learning a language, number sense takes practice, it requires frequent exposure, and is built up by drawing children's attention to numerical and mathematical ideas.

Specific activities to do at home include estimating and measuring (length, time, weight, volume, etc).

Make math a part of everyday life by asking questions about what you see around and asking them to find examples of the concepts they are currently learning.

3) Play games
We play a lot of games at school and ask the kids to play them at home with their parents as homework. The games don't go stale when we move on, parents can play old games again. Some games are very calculation heavy. These are great opportunities to flex the Concrete-Pictorial-Abstract muscles.

Other games (or explorations) are much more about strategy. These are a great place to practice the other questions, especially "what do you notice?" and "what do you wonder?"

Math Teachers at Play #100 - (Blog Carnival)

Wow, the 100th Math Teachers at Play! Such an honor to put together this milestone edition. Thanks to Denise Gaskins for creating and managing this great resource. Let her know if you are interested in hosting in the future.

I asked the 3J's for observations and facts about 100:

• It is written with a 1 and two 0s
• Square number (10 x 10)
• It is a sum of two squares 64 + 36
• It is a sum of two primes in several ways: 97 + 3, 89 + 11, 83 + 17, 71 + 29, 53 + 47
• 1100100 in binary
• 100 has 9 factors, which sounds like a lot, but is not an anti-prime
• 100 is the start of a 26 term Collatz Sequence: 100, 50, 25, 76, 38, 19, 58, 29, 88, 44, 22, 11, 34, 17, 52, 26, 13, 40, 20, 10, 5, 16, 8, 4, 2, 1 (little program for Collatz play)

Number Gossip revealed that it is a practical, powerful, happy, odious number. Hmm, a jolly dictator?

Here's a very familiar 100 in our daily life:

Unfortunately, this 100 makes far fewer appearances:

A 100 puzzle

To get started, here's a tricky puzzle that, semi-famously, has been a Google interview question. This phrasing comes from Good Riddles Now:

There are 100 prisoners lining up to go to jail. Each prisoner is wearing a hat that is either black or white. The prisoners don't know their own hat color, just the hat color of those in front of them in line (the first prisoner in line can't see anyone's hat and the last prisoner can see everyone's hat except their own). Starting from the back, one of the guards asks each prisoner what color their hat is. If they are correct they get to go free but if they are wrong they go to jail. 

If the prisoners get to discuss a plan, how can at least 99 of them be saved?

Talking about math
I blended ages here because I've noticed a lot of great cross-age pollination. The little ones are often very engaged and surprisingly insightful on topics that seem much more mature, while elementary conversations often touch some deep concepts.

AO Fradkin and her daughter discussed "how long is 3 minutes?" Hard to get deeper than the nature of time and how our perception depends on context (or does it?)

A view of Fermat's role in Fermat's Last Theorem from Mathematical Enchantments that nicely touching the fact of changing tastes in mathematical research. What mathematical preferences do you and your kids have?

Some tidbits for the Math is Everywhere meme:

1. Using big data to analyze story arcs.
2. Life through a Mathematician's Eyes has two posts on What a Mathematician should see in Amsterdam and Visiting Amsterdam like a Mathematician

I can never resist an icosahedron picture and there's a nice one in the first Amsterdam post:

Enjoy a joke anecdote about mathematical precision from Curiousa Mathematica. I like to tell elementary kids these kinds of jokes and see how they respond. It is a delight when they get it, but I also like to laugh at their deadpan expressions when they don't understand. 

Finally, a great way to start talking about math is to do math where you'll be seen doing it. This might require an emergency math kit (from Solve My Maths.)

Crafts and Constructions

Generally, these are accessible to young children, but also have some deep mathematics that offer something for any of us to explore.

Something our kids recently made: Flextangles craft activity (3d flexagons)

We love optical illusions and Sugihara's Illusion is a fantastic one. This post gives an explanation, then the next provides a printable. Agamographs are an accessible craft with a similar idea (instructions on Babble Dabble Do).

Benjamin Leis writes about an eye-catching decomposition and recomposition puzzle to start some exploration: hinged polygons.

Kira Zelbo offers up a free booklet that introduces isometric dot paper for drawing and visualizing three dimensional forms: Spatial Learning. Education Realist recently documented his experience using isometric grid paper with his class in Great Moments in Teaching.

Elementary Explorations and Middle School Mastery

Denise Gaskins (have you heard of her?) stimulates a discussion of favorite puzzle books in her review of Lilac Mohr's Math and Magic In Wonderland.

AO Fradkin again, helping some early elementary kids in discovering the triangle inequality.

John Golden serves another ace with his Wimbledon Game.

An old post from Sue Downing caught my attention: these place value cups are a great idea and make me think (fondly) of combination bike locks.

Addition Boomerang is a Mathpickle activity we played recently with our 1st-4th graders. When can you make 100? With that target, of course we had to include it in this MTaP!

Math Minds has ants-on-the-brain in 100 hungry ants. Trust me, it is better than ants in the pants.

Second graders explore proofs based on a geometric investigation: Squarable Numbers

Another post from Sue Downing that came in handy recently: "Is that all there are?" Multiplication facts. Now that you know the multiplication facts aren't scary, get some practice by playing with 

anti-primes (Numberphile). We got a lot of value out of seeking the anti-prime (er, highly composite number) that follows 24.

Number bracelet investigation. Our school and family have looked at this several times. Another great extension is to work in bases other than 10. Another way to understand it is working modulo 10 (or whatever integer you choose to set to 0).

Baseball, with its large collection of player and team stats, offers a natural entry point for mathematical conversations. Mashup Math walks through some examples in Mathematics of Major League Baseball. For a related take on this idea, see Mathpickle's Introducing Stats to Younger Children.

High School Adventures

Mrs E shares a lesson plan focused on getting kids to analyze and critique advertisements at Mrs E Teaches Math. An important life skill on its own and Mrs E sees it as a helpful bridge into writing proofs.

What happens when you are living inside a word problem? Math in real life.

I don't think this is a recent addition from Dan Meyer, but his Finals Week three-act seemed more fun to do during summer, away from the normal stresses of the school year.

There are some elementary and sophisticated ways to think about divisibility rules. In this post Curious Cheetah hits a bunch at one time.

"Stoichiometry is the math of chemistry," according to Amy Roediger. Here is her explanation and a discussion of teaching methods, parts 1, 2, and 3

Manan Shah contributes his approach to dealing with Annoying Function Notation. Can you make sense of these contrasting pairs:

Also, make sure to check out Manan's curation of the latest Carnival of Mathematics.

Po Shen Lo and Mike Lawler: a great combination... and Mike gives us two posts with PSL (first and second)!

Step into some difficult probability, statistics, and forecasting with Big Thompson Flood.

Singapore Maths Tuition walks us through a vector calculation to find the foot of a perpendicular from a point and to a line.

Ben Vitalis has a constant stream of interesting challenges, most accessible to algebra students: Odds equal Evens.

Puzzling Recreations

Math Arguments makes a surprising re-appearance to post a nice probability dice from Ben Orlin.

Lisa Winer (star of the 99th MTaP) talks about her plexer puzzles. Aside from being fun, these are a good place to practice Notice & Wonder.

Our family has recently become fans of the Futility Closet podcast, especially their lateral thinking puzzles.

Reminder: one great thing to do with any puzzle is CREATE YOUR OWN!!!

Teaching Tips

Which one doesn't belong (WODB) is a great format for a rich discussion. WODB.ca has a really nice collection of mathematical WODBs. I was reminded of this resource by this delightful WODB of WODB from John Golden.

Joe Schwartz continues a MTBoS theme of getting fixes for worksheets.

Amy Roediger put together a good collection of resources as she prepared to lead a

coding camp. A Recursive Process has a further discussion of the links between coding and math, including some more resources.

The folks at the Mind Research Institute have put together a summer reading list of 9 Enlightening Summer Reads for Math Teachers. The list mixes sci-fi and books about teaching. If you don't know the Mind Research Institute, they are behind/linked with ST Math, an on-line elementary grades math system that I really like. I had the opportunity to trial their system years ago and recently went through their free demo.  Now, if only someone there would be willing to get in touch and tell me how I could subscribe for my kids to use .....!

TMC16
Twitter Math Camp 2016 was just held and there are a lot of math teachers blogging about their experiences. If the posts I've gathered above aren't enough for you, I suggest hitting J Fairbanks's blog 8 is My Lucky Number for 10 (wow!) posts about the convention and further links.

Beast Academy and Dreambox (reviews)

Conflict of interest statement: I do not have a current or pending financial relationship with Art of Problem Solving, but I have several friends on their board and have had direct contact with several other people there. We purchased and currently own all of the books I review below.

I have no relationship with Dreambox. We tested the program using their free trial and then paid for a 6 month subscription.

---

Beast Academy

What is it?
Beast Academy (from Art of Problem Solving) is a book series with 10 "guidebooks" and 10 parallel "practice" books targeted to 3rd, 4th, and fifth graders. Note that the first of 4 books for fifth grade has only recently come out and they are planning to extend to 16 x 2 books covering 2nd to 5th. We do not have either 5A or 5B yet.

We have read all 8 books from 3a to 4d; J1 and J2 have gone through practice books 3A - 3C.

While these are not math exercise apps, I'm going to borrow some of the elements I've used in past app reviews. One key point I want to emphasize for both books and apps: the way you use them can also determine their benefits or costs.

TZB3
To drive this point home, let's start with Tracy Zager's Big Three criteria (see here):

1. No time pressure: Neutral since this is really up to you, parents.

Do you set a timer when they start a page of practice or a question? Do you require a certain amount of time spent on math practice? While the books do not suggest or impose a sense of time pressure, there are story segments involving math competitions that imply speed is important.

One time element that is and has always been great about physical books is that they sit around. This means they are available and tempting. Almost every day, there will be someone flipping open one of the BA guidebooks, even J3 for whom the material is too advanced right now.

2. Conceptual basis: yes (pass)

The books introduce models, contexts, and conceptual ways of considering problems and techniques. 

3. How are mistakes handled: again, this depends on you and your kids

My approach is to go through the problems and select ones to discuss. I don't use the answer key, so I do the problems myself. This means we have three categories of questions to discuss (a) answered correctly and I found interesting, (b) answered incorrectly, (c) answered correctly by the kid, but I made a mistake. 

Also, I am very positive in how I talk about mistakes. The key message is that these are actually the best learning opportunities and create a chance for us to understand our own thinking.

Preliminary summary: whether Beast Academy (or any printed material) passes the thresholds depends on how  you plan to use it. If you want to deviate from Tracy's guidelines, either adding time pressure or incentives based on minimizing mistakes, you probably should think carefully about whether that's wise.

The good

For my kids, the stories and themes in the guidebooks hit the right tone. They are engaging and funny, with a humor that is occasionally silly or corny. An extended quote from The Princess Bride certainly wins some extra points as well. More bonus points for becoming, via malapropism, the source of J3's current catch-phrase, "I get it: pointillism!"

For me, the organizing theme of the material seems to be "ideas you encounter when playing with math." In some cases, the exercises create "aha moments," like when J1 realized he didn't always have to calculate side lengths of a polygon to use his knowledge of its perimeter in a challenge. In other cases, like calculating (n+1) x (n-1) there are interesting patterns to notice and connections to make.

I'd note that the workbooks are absolutely essential as there is a lot of material that is introduced in the context of exercises. I think these books are excellent, well selected, well sequenced, with enough repetition to facilitate mastery and enough variation to avoid boredom. In fact, I really enjoy doing the problems myself.

Overall, we find the practice books an especially good source of cues for quick (5-15 minute) math conversations.

The Bad
Any worksheet-based system is weak in generating exploration and deeper investigation. Beast Academy partially addresses this by including open-ended games and an occasional investigation. While nice, this point remains a weakness. I don't want to belabor this point, since it is not a unique problem with Beast Academy. Indeed, I think it is a universal issue with static educational material.

Unfortunately, the only solution I know is to involve a human guide. Fortunately, I am able to play that role, asking their thoughts about interesting problems, helping them form connections with earlier or other material, getting them to follow useful side-branches or to continue more deeply into a particular area.

Eventually, of course, we hope to develop enough mathematical habits of mind that the kids will do these things on their own. Realistically, I don't think that will happen until they are well clear of any elementary age material!

The Ugly
I don't see any fatal flaws in Beast Academy.

Grand Summary
If you can use the material the way we do, I highly recommend Beast Academy.
If you can't or don't feel comfortable engaging as your kids' mathematical guide, these books are probably still one of the best options. Just don't set up a timer and demand perfect answers to all the questions!

Dreambox

Dreambox is a math facts, basic skills system. It has material from pre-school through high school. We have spent a lot of time with the elementary grade material and a little sampling of the high school content.

TZB3
Dreambox was one of Tracy Zager's positive examples in her app post, so we already expected it would pass these three criteria. After spending so much time with the system, though, we've seen that not all activities within DreamBox completely satisfy the checklist:

1. No time pressure
Some activities do include time pressure. For example, there are a family of "games" around multiplication automaticity where a collection of calculations stream across the screen. This really does raise the stress level for kids.

In a slightly different form, there are other activities involving virtual manipulatives that require the student to do something using the minimum number of moves. Like the time pressure, this seems to create confusion where the kids can get something right, but still get it wrong.

2. Conceptual Basis
I mostly concur with Tracy's original assessment. Almost all activities have a conceptual component. The timed calculations mentioned above don't, so those get a double demerit.

3. How errors are handled
Again, mostly agree with Tracy. However, there are some activities where, for a minor mistake, one is required to redo a number of manipulations, rather than fix the earlier work.

The good
The underlying math curriculum here is solid, if basic. The clear strength of this system is the pictorial representation of manipulatives offering models that build number sense, reflect operations, and show place value. In the early years section, where we have been spending most of our time, almost every activity is based around one of the manipulatives.

The other thing Dreambox does well is present a sensible progression for the different activity streams. I think this works especially well for J3 who is going through much of the material for the first time. As she encounters a new formulation, she will study it for a while and then there is a clear moment when she has figured out the new complication.

I'll give two examples. For J3, there is an activity to replicate a number bead pattern and then click the number of beads in the arrangement. Her primary tool is to count the beads one-by-one. In the most recent module, she gets a short view of the arrangement and then it is hidden (it can be revealed again, if you choose). This is forcing her to build new skills, either memorizing the arrangement to mentally count or a more advanced counting technique.

For J2, one of the place value exercises involves grouping items into pallets (1000s), cases (100s), boxes (10s) or loose items (1s). The current module asks him to consider multiple different ways to pack a given number. For example, 1385 items could be packed in 1 pallet, 3 cases, 8 boxes, and 5 loose items, or 13 cases and 85 loose items (among many other options).

One other strength of DreamBox is the email feedback to parents. Christopher Danielson recently noted this in a post: Parent Letters.


The Bad
I have seen three areas of weakness with Dreambox: the way mathematical tasks are presented, the pace of adaptive adjustment, and the absence of rich tasks. I'll talk about each of these in turn.

The theme gives an irritating appearance of choice. For example, in the early elementary section, the kids can play with dinosaurs, pirates, pixies, or animals. Under each of these, they have a further choice about what story to explore. Those choices, at least, lead them to different narratives and animated sequences.

At that point, all of the stories involve finding missing items. Users then see another choice asking where in 6 map regions they want to look for the missing items, but this isn't really a choice as there are no differences between regions and they will have to go through each region eventually.

Similar to Prodigy Game, the math tasks are presented as an annoyance to be overcome, the cost the student has to pay to move on with the story. Again, I find this creates unfortunate subtext to the mathematical experience.

Second, the adaptive adjustment is very slow, if it actually exists. In their FAQ, I see that they get questions about how to increase the challenge level, so this seems to be a common experience. Part of the problem is that they intentionally start students with material below their grade level.

Finally, the tasks in Dreambox are basic. While they may present a challenge for a new learner, as J3 is experiencing, they should eventually become so easy that they are boring. In some way, this feels like learning to solve math class tasks without having to develop or use any mathematical habits of mind.  Further, the thrill and fun of playing Dreambox lies in unlocking the animated stories and collecting tokens, not in doing math.

For J1 and J2, this thrill has worn off after about 2 months with the system.

The ugly
Nothing in Dreambox is a show-stopper.

Summary
Properly understood as a basic curriculum substitute or source of practice exercises, Dreambox is a solid application. Just don't make the mistake of thinking it will either foster a love of math nor deeper mental habits.

*Update* A quick comparison with ST Math
I was sitting on this review, partially written, for a long time. One thing that got me to finalize the review was going through the demo challenges on ST Math with J2. We had previously tested ST Math many years ago with J1 and it was really good. Once again, this is what I saw with J2: really cleverly presented scenarios that gave us good models for the math and a really fun user experience. After playing for about 30 minutes, J2 said: "this is a lot more fun than DreamBox."

If I can get a subscription, we'll test it more extensively and write a review to see whether that really holds up.

Wimbledon game

We have been playing the game Wimbledon from John Golden. J1 and J2 absolutely love the game and J3 has enjoyed pretending to play as well. Definitely try it out!

Below is a session report sharing some of our experiences with the game, including some alternative rules (aka mis-reading).

Our basic play
For the serve, we allow the following options:
(1) serve a single card from the top of the deck
(2) serve one or two cards from your own hand

We also allow returns that have the same value, if the largest card in the combination is higher. For example, 8 + 2 can be played on top of 7 + 3.

When playing doubles, we followed tennis conventions: one player serves the whole game, return of serve alternates. For the return of serve, the designated player must return on their own. For other returns, either of the partners alone or in combination can play cards for a return.

Having gone back to John's original post, I now see that we played with the inverse rules for aces. On the serve, we counted them as 11, all other times 1. We didn't distinguish between aces played from the hand or served from the deck, those were all 11s. That formed a strong advantage for the servers, while making aces essentially worthless for all other players.

With three players, I had the kids play as partners and I played with a ghost partner. The ghost partner would contribute cards randomly. When the ghost played cards that weren't large enough to be legal plays, we considered that an unforced error and awarded the point to the other team. While the ghost was able to hold serve for one game, it was a big disadvantage. An alternative for three players would be to have the ghost partner with the server and for everyone to take turns serving. This would put the server advantage (with our "house rules" for aces) against the ghost disadvantage.

A modification
In our play, we have found that the 10 value cards and aces (on serve) dominate game play. Here are two ideas to address that:

• Assign face cards values 11 for Jack, 12 for Queen, and 13 for King. Ace, on serve, can have a value 14.
• Allow players to combine as many cards as they like. This would probably work best with our "Further extension" rules below. A possible sub-variant is to only allow gradual escalation where the players can step from single card plays to 2 card plays, from 2 to 3, etc, but could not jump from a single card play to a 3 (or more) card play.

My instinct is that the variation we will like the most is to differentiate the face cards and allow gradual escalation for multi-card plays.

Further extension
Now that we've gotten comfortable with the basic and doubles games, we are considering a more complex version. The idea we are considering is to somehow limit the players' abilities to refresh their hands by redrawing so that burning a lot of cards will have a cost.

This is the rule modification, written for a 2 person game:

1. Create a draw pile for each player with 15 cards.
2. At the start of the game, each player draws 5 cards into their hand.
3. Points are played as in the normal rules
4. At the end of a point, the players refresh their hand up to 5 cards from their draw pile
5. If a player runs out of cards in their draw pile, they cannot draw additional cards to refresh their hand.
6. If both players run out of cards in their draw pile, then shuffle the pile of face-up played cards and give each player a new draw pile with 10 cards.
7. Repeat step 6 as often as needed

The idea of this variation is to thematically mimic the idea that one player could push too hard, too fast, and get tired out relative to the other player. Strategically, our idea is that this will also create a tension between dumping your own low cards and letting the opponent dump.

Humbling Improv

Last night, I was part of an improv comedy show. Probably my first time performing on stage for ... at least 25 years.

How did I do? A lot of room for improvement. Not at all a surprise, but what really sticks with me is that the mistakes I made were in the absolute basics of improv:

1. Listen to the other players
2. Accept
3. Who where what

Messing these up is common for beginning players and comes from a desperation to get laughs. I thought I was immune. I didn't think I was desperate. Heck, I didn't even care about the audience reaction.

But still, I made those mistakes and played badly. The experience was an interesting opportunity for better self-understanding and a vivid reminder to focus on the basics.

It was a great chance to fail and learn.

Am I dreaming?

Three Js playing cards together. Kid-initiated, kid-managed, no parents involved in any role:

Then, the game devolves a bit, based on excitement about a new set of library books that mommy has gotten:

Evens/odds and a quick update

Early years math seems to put a strange emphasis on even and odd numbers. Recently, a friend asked whether there was a point to this. Maybe it is just one of those little bits of terminology that we are asked to memorize for no reason?

By chance, this was something I had started considering about a month ago. It did seem strange that we spend so much time on this simple way of splitting integers. I wondered if it was worth the attention. From that point, my awareness was raised and I started noticing where it occurs and ways it links with more advanced concepts and future learning. My conclusion is that even/odd is surprisingly deep.

First, it is a simple version of concepts that will be developed further. For example, the alternating (starting with 0) even, odd, even,odd, even... is an illustration of a pattern. They will soon see other alternating patterns, then more complicated patterns and 2d or 3d patterns.

For another example, evens are multiples of 2, odds are numbers with a non-zero remainder when dividing by 2. This leads to understanding other multiple families, division, and division with remainder.

Second, the even/odd distinction is helpful for improved understanding of different calculations. For example, the observations that even+even = even, while odd + odd = even, etc. These can be used to help self-check their calculation and also will form early experiences with algebra. Similarly,
even x odd vs odd x odd reinforce understanding of multiplication. Again, this gets broadened for multiples of 3, 4, 5, etc.

Third, there are a lot of more advanced results that are easiest to prove by parity arguments. Sometimes we are working with a set of things that are even and the key observation is we can pair them up. Other times, we have a set that is odd and the key insight is that, when we pair them, one must be left over.

Recently, with the J1 and J2, we were looking at some constrained ways to put the numbers 1 to 25 on a 5x5 checkerboard. They were able to prove that some versions were impossible simply because 25 is odd, so there are more odd integers in 1 to 25 than even integers.

Lastly, there are techniques in computer science that involve even vs odd. This comes up pretty naturally because of the essential use of binary.

Some games

Recently, J1 and J2 have gotten hooked on some classic card games. In particular, we've been playing a lot of 3-handed cribbage. It is a nice way to do some simple addition practice and build intuition about probability. Probability is now getting even more share of mind: in the last couple of days we started playing poker together. This was actually inspired by some of our reading together.

We are reading the Pushcart War.

In one scene, there is a poker game. Of course, the J's insisted that I explain the game and were eager to try it out. J3 was the huge winner tonight, while I busted out. Oh well.